Question

A worker wants to load a 12 kg crate into a truck by sliding the crate up a straight ramp which is 2.5 m long and which makes an angle of 30 degrees with the horizontal. The worker believes that he can get the crate to the very top of the ramp by launching it at 5 m/s at the bottom and letting go. But friction is not neglible; the crate slides 1.6 m upthe ramp, stops, and slides back down.

Required:
a. Assuming that the friction force actingon the crate is constant, find its magnitude.
b. How fast is teh crate moving when it reachesthe bottom of the ramp?

Answer 1

a) The magnitude of the friction force is 55.851 newtons, b) The speed of the crate when it reaches the bottom of the ramp is 2.526 meters per second.

Step-by-step explanation:

a) This situation can be modelled by the Principle of Energy Conservation and the Work-Energy Theorem, where friction represents the only non-conservative force exerting on the crate in motion. Let consider the bottom of the straight ramp as the zero point. The energy equation for the crate is:

`U_(g,1)+K_(1) = U_(g,2)+K_(2)+ W_(fr)`

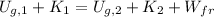
Where:

`U_(g,1)`,
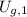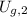`U_(g,2)` - Initial and final gravitational potential energy, measured in joules.

`K_(1)`,
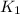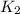`K_(2)` - Initial and final translational kinetic energy, measured in joules.

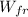`W_(fr)` - Work losses due to friction, measured in joules.

By applying the defintions of translational kinetic and gravitational potential energies and work, this expression is now expanded:

`m\cdot g \cdot y_(1) + (1)/(2)\cdot m\cdot v_(1)^(2) = m\cdot g \cdot y_(2) + (1)/(2)\cdot m\cdot v_(2)^(2) + \mu_(k)\cdot m \cdot g \cdot \cos \theta`

Where:

`m` - Mass of the crate, measured in kilograms.

`g` - Gravitational acceleration, measured in meters per square second.

`y_(1)`,
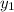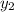`y_(2)` - Initial and final height of the crate, measured in meters.

`v_(1)`,
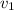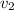`v_(2)` - Initial and final speeds of the crate, measured in meters per second.

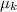`\mu_(k)` - Kinetic coefficient of friction, dimensionless.

`\theta` - Ramp inclination, measured in sexagesimal degrees.

The equation is now simplified and the coefficient of friction is consequently cleared:

`y_(1)-y_(2)+(1)/(2\cdot g)\cdot (v_(1)^(2)-v_(2)^(2)) = \mu_(k)\cdot \cos \theta`

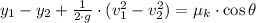
`\mu_(k) = (1)/(\cos \theta) \cdot \left[y_(1)-y_(2)+(1)/(2\cdot g)\cdot (v_(1)^(2)-v_(2)^(2)) \right]`

The final height of the crate is:

`y_(2) = (1.6\,m)\cdot \sin 30^(\circ)`

`y_(2) = 0.8\,m`

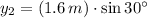
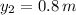
If
`\theta = 30^(\circ)`,
`y_(1) = 0\,m`,
`y_(2) = 0.8\,m`,
`g = 9.807\,(m)/(s^(2))`,
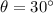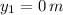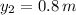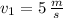`v_(1) = 5\,(m)/(s)` and
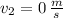`v_(2) = 0\,(m)/(s)`, the coefficient of friction is:

`\mu_(k) = (1)/(\cos 30^(\circ))\cdot \left\{0\,m-0.8\,m+(1)/(2\cdot \left(9.807\,(m)/(s^(2)) \right))\cdot \left[\left(5\,(m)/(s) \right)^(2)-\left(0\,(m)/(s) \right)^(2)\right] \right\}`

`\mu_(k) \approx 0.548`

Then, the magnitude of the friction force is:

`f =\mu_(k)\cdot m\cdot g \cdot \cos \theta`

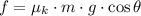
If
`\mu_(k) \approx 0.548`,
`m = 12\,kg`,
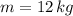`g = 9.807\,(m)/(s^(2))` and
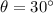`\theta = 30^(\circ)`, the magnitude of the force of friction is:

`f = (0.548)\cdot (12\,kg)\cdot \left(9.807\,(m)/(s^(2)) \right)\cdot \cos 30^(\circ)`

`f = 55.851\,N`

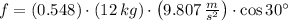
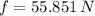
The magnitude of the force of friction is 55.851 newtons.

b) The energy equation of the situation is:

`m\cdot g \cdot y_(1) + (1)/(2)\cdot m\cdot v_(1)^(2) = m\cdot g \cdot y_(2) + (1)/(2)\cdot m\cdot v_(2)^(2) + \mu_(k)\cdot m \cdot g \cdot \cos \theta`

`y_(1)+(1)/(2\cdot g)\cdot v_(1)^(2) =y_(2) + (1)/(2\cdot g)\cdot v_(2)^(2) + \mu_(k)\cdot \cos \theta`

Now, the final speed is cleared:

`y_(1)-y_(2)+ (1)/(2\cdot g)\cdot v_(1)^(2) -\mu_(k)\cdot \cos \theta= (1)/(2\cdot g)\cdot v_(2)^(2)`

`2\cdot g \cdot (y_(1)-y_(2)-\mu_(k)\cdot \cos \theta) + v_(1)^(2) = v_(2)^(2)`

`v_(2) = \sqrt{2\cdot g \cdot (y_(1)-y_(2)-\mu_(k)\cdot \cos \theta)+v_(1)^(2)}`

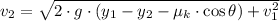
Given that
`g = 9.807\,(m)/(s^(2))`,
`y_(1) = 0.8\,m`,
`y_(2) = 0\,m`,
`\mu_(k) \approx 0.548`,
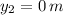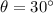`\theta = 30^(\circ)` and
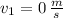`v_(1) = 0\,(m)/(s)`, the speed of the crate at the bottom of the ramp is:

`v_(2)=\sqrt{2\cdot \left(9.807\,(m)/(s^(2)) \right)\cdot [0.8\,m-0\,m-(0.548)\cdot \cos 30^(\circ)]+\left(0\,(m)/(s) \right)^(2)}`

`v_(2)\approx 2.526\,(m)/(s)`

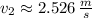
The speed of the crate when it reaches the bottom of the ramp is 2.526 meters per second.