Question

Thomas Kratzer is the purchasing manager for the headquarters of a large insurance company chain with a central inventory operation.​ Thomas's fastest-moving inventory item has a demand of 5,900 units per year. The cost of each unit is ​$102​, and the inventory carrying cost is ​$9 per unit per year. The average ordering cost is ​$29 per order. It takes about 5 days for an order to​ arrive, and the demand for 1 week is 118 units.​ (This is a corporate​ operation, and there are 250 working days per​ year).

Required:
a. What is the EOQ?
b. What is the average inventory if the EOQ is used?
c. What is the optimal number of orders per year?
d. What is the optimal number of days in between any two orders?
e. What is the annual cost of ordering and holding inventory?
f. What is the total annual inventory cost, including cost of the 6,000 units?

Answer 1

Answer: Please find answers below

Step-by-step explanation:

(a) Economic order quantity EOQ =
`√(2 X Annual Demand X Ordering Cost) / Carrying Cost))`

=
`√(2 X 5,900 X 29 / 9 )` =
`√(38,022.222)`

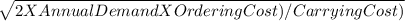
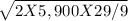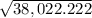
= 194.99 units

(b) Average number of units = Economic order quantity / 2

= 194.99 / 2

= 97.496 units

(c) Optimal number of orders = Annual Demand / Economic order quantity

= 5,900units / 194.99 units =30.26

(d) Optimal number of days between two orders = Number of working days / Optimal number of orders

= 250 days / 30.26

= 8.26

Total ordering cost = Cost per order X Number of orders

= $29 X 30.26

= $ 877.54

Total holding cost = Average inventory X carrying cost per unit

= 194.99 /2 X $9

= $877.455

(e) Annual cost of ordering and holding inventorY =Total ordering cost + Total carrying cost

= $ 877.54 + $877.455

= $ 1,754.995 ≈ $1,755

(f) Total annual inventory cost =Total ordering cost +Total holding cost + Actual cost of 5900 units at $102 per unit

= $ 877.54 + $877.455 + (5,900 x 102) = $1754.995 +601,800= $603,554.995≈$603,555

Total annual inventory cost =Total ordering cost +Total holding cost + Actual cost of 6000 units at $102 per unit

= $ 877.54 + $877.455 + (6000 x 102) = $1754.995 +612,000= $613,754.995≈$613,755