Question

Bob Nale is the owner of Nale's Texaco GasTown. Bob would like to estimate the mean number of litres (L) of gasoline sold to his customers. Assume the number of litres sold follows the normal distribution with a standard deviation of 18 L. From his records, he selects a random sample of 18 sales and finds the mean number of litres sold is 56.

a. What is the point estimate of the population mean? (Round the final answer to the nearest whole number.)


The point estimate of the population mean is
litres.


b. Develop a 80% confidence interval for the population mean. (Round the final answers to 3 decimal places.)


The 80% confidence interval for the population mean is between
and
.


c. Interpret the meaning of part (b).


If 100 such intervals were determined, the population
mean
would be included in about
intervals.

Answer 1

a

The point estimate of the population mean is
`\= x = 56`

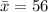
b

The 80% confidence level is
`50.57 < \mu < 61.43`

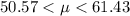
c

There is 80% confidence that the true population mean lies within the confidence interval.

Explanation:

From the question we are told that

The sample size is n = 18

The standard deviation is
`\sigma = 18 \ L`

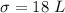
The sample mean is
`\= x = 56`

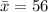
Generally the point estimate of the population mean is equivalent to the sample mean whose value is
`\= x = 56`

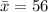
Given that the confidence interval is 80% then the level of significance is mathematically represented as

`\alpha = 100 - 80`

`\alpha = 20 \%`

`\alpha = 0.20`

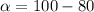
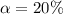
Next we obtain the critical value of
`(\alpha )/(2)` from the normal distribution table

The value is
`Z_{( \alpha )/(2) } = 1.28`

Generally the margin of error is mathematically evaluated as

`E = Z_{(\alpha )/(2) } * (\sigma )/(√(n) )`

=>
`E = 1.28 * (18 )/(√(18) )`

=>
`E = 5.43`

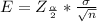
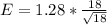
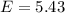
Generally the 80% confidence interval is mathematically represented as

`\= x - E < \mu < \= x + E`

=>
`56 - 5.43 < \mu < 56 + 5.43`

=>
`50.57 < \mu < 61.43`

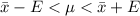
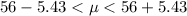
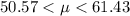
The interpretation is that there is 80% confidence that the true population mean lies within the limit