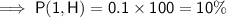Question

Hi can someone help ​

Answer 1

P(1, H) = 1/10 = 0.1 = 10%

Explanation:

`\sf Probability\:of\:an\:event\:occurring = (Number\:of\:ways\:it\:can\:occur)/(Total\:number\:of\:possible\:outcomes)`

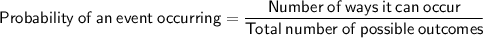
From the given table, we can see that there are 10 possible outcomes.

There is only one way that (1, H) can occur.

Therefore:

`\sf \implies P(1, H)=(1)/(10)`

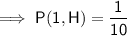
To write the probability as a decimal, divide 1 by 10. To divide any number by 10, move the decimal point one place to the left:

`\implies \sf P(1,H) =(1)/(10)=0.1`

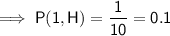
To write the probability as a percentage, multiply by 100.

To multiply by 100, move the decimal point 2 places to the right:

`\implies \sf P(1,H) = 0.1 * 100 = 10\%`

Answer 2

• 1/10 or 10%

Explanation:

The number of outcomes is 10.

There is one outcome with (1, H).

Required probability is:

• P(1, H) = 1/10 or 0.1 or 10%