Question

Find the remainder in the Taylor series centered at the point a for the following function. Then show that limn→[infinity]Rn(x)=0 for all x in the interval of convergence.

f(x)=cos x, a= π/2

Answer 1

`|R_n (x)| \leq (|x - (\pi)/(2)|^(n+1))/((n+1)!)`

Explanation:

From the given question; the objective is to show that :

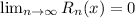`\lim_(n \to \infty) R_n (x) = 0` for all x in the interval of convergence f(x)=cos x, a= π/2

Assuming for the convergence f the taylor's series , f happens to be the derivative on an open interval I with a . Then the Taylor series for the convergence of f , for all x in I , if and only if
`\lim_(n \to \infty) R_n (x) = 0`

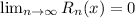
where;

`\mathtt{R_n (x) = (f^((n+1)) (c))/(n+1!)(x-a)^(n+1)}`

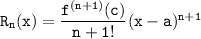
is a remainder at x and c happens to be between x and a.

Given that:

a= π/2

Then; the above equation can be written as:

`\mathtt{R_n (x) = (f^((n+1)) (c))/(n+1!)(x-(\pi)/(2))^(n+1)}`

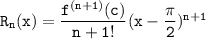
so c now happens to be the points between π/2 and x

If we recall; we know that:

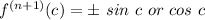`f^((n+1))(c) = \pm \ sin \ c \ or \ cos \ c` (as a result of the value of n)

However, it is true that for all cases that
`|f ^((n+1)) \ (c) | \leq 1`

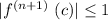
Hence, the remainder terms is :

`|R_n (x)| = | (f^((n+1))(c))/((n+1!))(x-(\pi)/(2))^(n+1)| \leq (|x - (\pi)/(2)|^(n+1))/((n+1)!)`

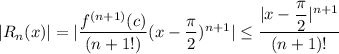
If
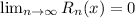`\lim_(n \to \infty) R_n (x) = 0` for all x and x is fixed, Then

`|R_n (x)| \leq (|x - (\pi)/(2)|^(n+1))/((n+1)!)`