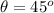Question

A projectile has a range of 60 m and can reach a maximum height of 15 m. Calculate the angle at which projectile is fired? ( g = 10m/s2 )

Answer 1

The angle the projectile was fired is
`45^o`

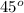
Step-by-step explanation:

Recall the formulas for maximum height and ranges for a projectile fired with initial velocity "v" at an angle
`\theta`:

`h = (v^2\,sin^2(\theta))/(2\,g)\\R=(v^2\,sin(2\,\theta))/(g)`

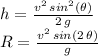
we can use them to solve for the angle by first isolating the value
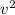`v^2` which is common in both equations:

`v^2=2\,h\,g/sin^2(\theta)=2\,(15)\,g/sin^2(\theta)=30\,(g)/sin^2(\theta) \\ \\v^2=R\,g/sin(2\,\theta)=60\,(g)/sin(2\,\theta)`

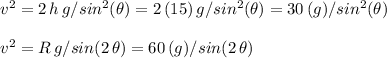
and now, making those to expressions equal and using the formula for the sine of a double angle, we get:

`(30\,(9.8))/(sin^2(\theta)) =(60\,(g))/(sin(2\,\theta)) \\30\,(g)\,sin(2\,\theta)=60\,(g)\,sin^2(\theta)\\sin(2\,\theta)=2\,sin^2(\theta)\\2\,sin(\theta)\,cos(\theta)=2\,sin(\theta)\,sin(\theta)\\cos(\theta)=sin(\theta)`

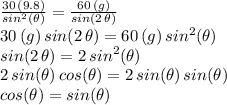
This happens when
`\theta=45^o`