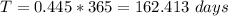Question

In the summer of 2010 a huge piece of ice roughly four times the area of Manhattan and 500 m thick caved off the Greenland mainland.

Required:
a. How much heat would be required to melt this iceberg (assumed to be at 0°C) into liquid water at 0°C?
b. The annual U.S. energy consumption is 1.2 x 10^20 J. If all the U.S. energy was used to melt the ice, how many days would it take to do so?

Answer 1

a

`Q = 5.34 *10^(19) \ J`

b

`T = 0.445 * 365 = 162. 413 \ days`

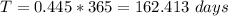
Step-by-step explanation:

From the question we are told that

The area of Manhattan is
`a_k = 87.46 *10^(6) \ m^2`

The area of the ice is
`a_i = 4* 87.46 *10^(6 ) = 3.498 *10^(8)\ m^2`

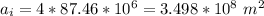
The thickness is
`t = 500 \ m \\`

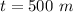
Generally the volume of the ice is mathematically represented is

`V = a_i * t`

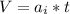
substituting value

`V = 500 * 3.498*10^(8)`

`V = 1.75 *10^(11)\ m^3`

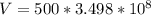
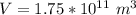
Generally the mass of the ice is

`m_i = \rho_i * V`

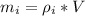
Here
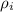`\rho_i` is the density of ice the value is
`\rho _i = 916.7 \ kg/m^3`

=>
`m_i = 916.7 * 1.75*10^(11)`

=>
`m_i = 1.60 *10^(14) \ kg`

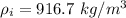
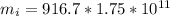
Generally the energy needed for the ice to melt is mathematically represented as

`Q = m _i * H_f`

Where
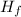`H_f` is the latent heat of fusion of ice and the value is
`H_f = 3.33*10^(5) \ J/kg`

=>
`Q = 1.60 *10^(14) * 3.33*10^(5)`

=>
`Q = 5.34 *10^(19) \ J`

Considering part b

We are told that the annual energy consumption is
`G = 1.2*10^(20 ) \ J / year`

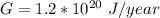
So the time taken to melt the ice is

`T = ( 5.34 *10^(19))/( 1.2 *10^(20))`

`T = 0.445 \ years`

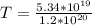
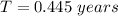
converting to days

`T = 0.445 * 365 = 162. 413 \ days`