Answer:
The null hypothesis is rejected and research hypotheses is supported
Explanation:
From the question we are told that
The population mean is
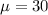
The standard deviation is
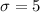
The sample size is n = 1
The cutoff Z score for significance is

The mean score is
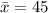
Generally the test hypothesis is mathematically represented as
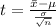
=>
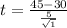
=>
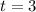
From the obtained value we can see that
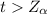
Hence the null hypothesis is rejected and research hypotheses is supported