Question

Based on experience, the Ball Corporation’s aluminum can manufacturing facility in Ft. Atkinson, Wisconsin, knows that the metal thickness of incoming shipments has a mean of 0.2771 mm with a standard deviation of 0.000855 mm.

(a) A certain shipment has a diameter of 0.2742. Find the standardized z-score for this shipment. (Round your answer to 3 decimal places.)


z



(b) Is this an outlier?


Yes

No

Answer 1

(a) The standardized z-score for this shipment is -3.392.

(b) Yes, this an outlier.

Explanation:

We are given that the Ball Corporation’s aluminum can manufacturing facility in Ft. Atkinson, Wisconsin, knows that the metal thickness of incoming shipments has a mean of 0.2771 mm with a standard deviation of 0.000855 mm.

Let X = the metal thickness of incoming shipments.

The z-score probability distribution for the normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = mean thickness = 0.2771 mm

`\sigma` = standard deviation = 0.000855 mm

(a) Now, it is given that a certain shipment has a diameter of 0.2742 mm and we have to find the standardized z-score for this shipment.

So, z-score =
`(X-\mu)/(\sigma)`

=
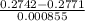`(0.2742-0.2771)/(0.000855)` = -3.392

Hence, the standardized z-score for this shipment is -3.392.

(b) Yes, we can consider this as an outlier because the standardized z-score is very large and this value is far from the population mean.