Question

Listed below are numbers of Internet users per 100 people and numbers of scientific award winners per 10 million people for different countries. Construct a​ scatterplot, find the value of the linear correlation coefficient​ r, and find the​ P-value of r. Determine whether there is sufficient evidence to support a claim of linear correlation between the two variables. Use a significance level of α= 0.01.

Internet Users 80.3 78.2 56.4 67.6 77.7 38.6

Award Winners 5.6 9.3 3.2 1.6 10.9 0.1


Required:

a. Construct a scatterplot.

b. Determine the null and alternative hypotheses.

c. The test statistic is:_________

d. The P-value is:_________

Answer 1

There is not sufficient evidence to support a claim of linear correlation between the two variables.

Explanation:

(a)

The scatter plot for the provided data is attached below.

(b)

The hypothesis to test significance of linear correlation between the two variables is:

H₀: There is no linear correlation between the two variables, i.e. ρ = 0.

Hₐ: There is a significant linear correlation between the two variables, i.e. ρ ≠ 0.

(c)

Use the Excel function: =CORREL(array1, array2) to compute the correlation coefficient, r.

The correlation coefficient between the number of internet users and the award winners is,

r = 0.786.

The test statistic value is:

`t=r\sqrt{(n-2)/(1-r^(2))}`

`=0.786*\sqrt{(6-2)/(1-(0.786)^(2))}\\\\=2.5427\\\\\approx 2.54`

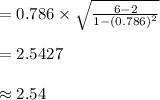
Thus, the test statistic is 2.54.

(d)

The degrees of freedom is,

df = n - 2

= 6 - 2

= 4

Compute the p-value as follows:

`p-value=2\cdot P(t_(n-2)<2.54)=2* 0.032=0.064`

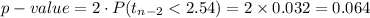
*Use a t-table.

p-value = 0.064 > α = 0.05

The null hypothesis will not be rejected.

Thus, it can be concluded that there is not sufficient evidence to support a claim of linear correlation between the two variables.