Answer:
2.25.
Explanation:
x^2 + 3x - 18 = 0
First, we need to write the constant on the right of the equation. So, we add 18 to both sides.
x^2 + 3x = 18.
Now, we find the number that will complete the square. It will be
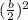 .
.
In this case, b = 3.
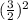
= (1.5)^2
= 2.25.
So, the number that will complete the square to solve the equation is 2.25, or 2 and 1/4, or 9/4.
Hope this helps!