Question

What best explains whether a triangle with side links 5 cm 13 cm and 12 cm is a right triangle

Answer 1

Pythagorean theorem

Explanation:

We can explain it using the Pythagorean theorem. Right triangles always have a hypotenuse which is the longest side. That means 13 must be the hypotenuse of the triangle. The Pythagorean theorem is a^2+b^2=c^2

We already know all the values since every side is given so we just fill it in.

5^2+12^2=13^2

25+144=169

169=169

It is a right triangle

Answer 2

Explanation:

Pythagoras Theorem

If the sum of the squares of the smaller two sides is equal to the square if the third side then it is a right triangle

`{a}^(2) + {b}^(2) = {c}^(2)`

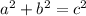
So, (5)^2 + (12)^2

is 25 + 144 = 169

Which is equal to (13)^2 which is also 169

The sides of the given triangle follows pythagoras theorem, therefore it is a right triangle

Hope it helps:)