Question

Please help ASAP!! question is in the image below

Answer 1

(a) ∠EFH = 68°

(b) ∠EGF = 21°

Explanation:

(a) The given parameters are;

The diameter of the circle = Segment
`\overline{EG}`

Arc
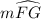`m\widehat{FG}` = 138°

∠GFH = 22°

∠ EFG = (Angle subtended by the diameter EG at the center)

Arc mEG = 180° (Arc subtended by the diameter of a circle = 180°)

∠ EFG is subtended by the diameter EG at the center

∴ ∠ EFG = 90° (Angle at the center = 2 times angle at the circumference)

∠EFG = ∠EFH + ∠GFH (Angle addition postulate)

∴ ∠EFH = ∠EFG - ∠GFH = 90° - 22° = 68°

∠EFH = 68°

(b) ∠EGF

Arc GH = 44°

Arc mFE + Arc
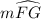`m\widehat{FG}` + Arc mEHG = 360 (Sum of angles at the center of a circle)

Arc mFE = 360 - ( Arc
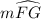`m\widehat{FG}` + Arc mEHG )

Arc mFE = 360 - 180 - 138 = 42°

∠EGF = Arc mFE/2 (Angle at the center = 2 times angle at the circumference)

∠EGF = 42/2 = 21°

∠EGF = 21°