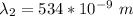Question

In a double-slit experiment, light from two monochromatic light sources passes through the same double slit. The light from the first light source has a wavelength of 587 nm. Two different interference patterns are observed. If the 10th order bright fringe from the first light source coincides with the 11th order bright fringe from the second light source, what is the wavelength of the light coming from the second monochromatic light source?

Answer 1

The wavelength is
`\lambda_2 = 534 *10^(-9) \ m`

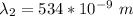
Step-by-step explanation:

From the question we are told that

The wavelength of the first light is
`\lambda _ 1 = 587 \ nm`

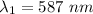
The order of the first light that is being considered is
`m_1 = 10`

The order of the second light that is being considered is
`m_2 = 11`

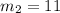
Generally the distance between the fringes for the first light is mathematically represented as

`y_1 = ( m_1 * \lambda_1 * D)/(d)`

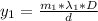
Here D is the distance from the screen

and d is the distance of separation of the slit.

For the second light the distance between the fringes is mathematically represented as

`y_2 = ( m_2 * \lambda_2 * D)/(d)`

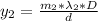
Now given that both of the light are passed through the same double slit

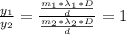
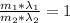
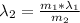
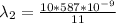
`(y_1)/(y_2) = ((m_1 * \lambda_1 * D)/(d) )/((m_2 * \lambda_2 * D)/(d) ) = 1`

=>
`( m_1 * \lambda _1 )/( m_2 * \lambda_2) = 1`

=>
`\lambda_2 = (m_1 * \lambda_1)/(m_2)`

=>
`\lambda_2 = (10 * 587 *10^(-9))/(11)`

=>
`\lambda_2 = 534 *10^(-9) \ m`