1 Answer

Verbanicm · Answer 1 · 2021-01-24T00:14:28+0000

Answer:

The probability that none of the LED light bulbs are defective is 0.7374.

Explanation:

The complete question is:

What is the probability that none of the LED light bulbs are defective?

Solution:

Let the random variable X represent the number of defective LED light bulbs.

The probability of a LED light bulb being defective is, P (X) = p = 0.03.

A random sample of n = 10 LED light bulbs is selected.

The event of a specific LED light bulb being defective is independent of the other bulbs.

The random variable X thus follows a Binomial distribution with parameters n = 10 and p = 0.03.

The probability mass function of X is:

$P(X=x)={10\choose x}(0.03)^(x)(1-0.03)^(10-x);\ x=0,1,2,3...$

Compute the probability that none of the LED light bulbs are defective as follows:

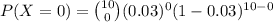
$P(X=0)={10\choose 0}(0.03)^(0)(1-0.03)^(10-0)$

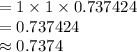
$=1* 1* 0.737424\\=0.737424\\\approx 0.7374$

Thus, the probability that none of the LED light bulbs are defective is 0.7374.

Please log in or register to add a comment.