Question

Sandra spotted the sailboat from the shore and measured the angle from the waterline to the top of the boats mast to be 7° if the top of the mask is 23 feet above the water how far is the middle of the sailboat from the shore? Estimate your answer to the nearest tenth.

Answer 1

The middle of the sailboat is approximately 268.8 feet from the shore.

Explanation:

Let the distance from shore to the middle of the boat be represented by x, the angle of elevation of Sandra from the shore to the top of the boat mast is 7°. Applying the required trigonometric function to this question, we have;

Tan θ =
`(opposite)/(adjacent)`

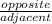
Tan 7° =
`(23)/(x)`

⇒ x =
`(23)/(Tan 7^(0) )`

=
`(23)/(0.12279)`

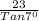
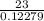
= 268.7515

∴ x = 268.8 feet

The middle of the sailboat is approximately 268.8 feet from the shore.