Question

The 4th term of an exponential sequence is 108 and the common ratio is 3. Calculate the value of the eighth term of the sequence.

Answer 1

The eighth term is 8748

Explanation:

Since the sequence is a geometric sequence

For an nth term in a geometric sequence

`A (n) = a ({r})^(n - 1)`

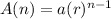
where

a is the first term

r is the common ratio

n is the number of terms

To find the eighth term we must first find the first term

4th term = 108

common ratio = 3

That's

`A(4) = a ({r})^(4 - 1)`

`108 = a ({3})^(3)`

`27a = 108`

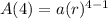
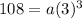
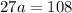
Divide both sides by 27

a = 4

The first term is 4

For the eighth term

`A(8) = 4 ({3})^(8 - 1)`

`A(8) = 4({3})^(7)`

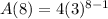
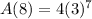
The final answer is

A(8) = 8748

The eighth term is 8748

Hope this helps you