Question

Find the​ mean, variance, and standard deviation of the binomial distribution with the given values of n and p. ​, The​ mean, ​, is nothing. ​(Round to the nearest tenth as​ needed.)

Answer 1

Complete Question

Find the​ mean, variance, and standard deviation of the binomial distribution with the given values of n and p. ​, The​ mean, ​, is nothing. ​(Round to the nearest tenth as​ needed.)

p = 0.6 n = 18

Answer:

The mean
`\mu = 10.5`

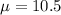
The standard deviation
`\sigma = 2.08`

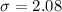
The variance
`var = 4.32`

Explanation:

From the question we are told that

The probability of success is
`p = 0.6`

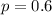
The sample size is
`n = 18`

Generally given that the distribution is binomial, then the probability of failure is mathematically represented as

`q = 1- p`

substituting values

`q = 1- 0.6`

`q =0.4`

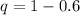
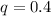
Generally the mean is mathematically evaluated as

`\mu = np`

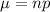
substituting values

`\mu = 18 * 0.6`

`\mu = 10.5`

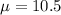
The standard deviation is evaluated as

`\sigma = √(npq)`

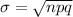
substituting values

`\sigma = √(18 * 0.6 * 0.4)`

`\sigma = 2.08`

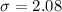
The variance is evaluated as

`var = \sigma^2`

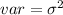
substituting value

`var = 2.08^2`

`var = 4.32`