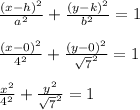Question

Find the standard form of the equation of the ellipse with the given characteristics. center: (0, 0) focus: (3, 0) vertex: (4, 0)

Answer 1

`(x^2)/(4^2)+(y^2)/(√(7) ^2)=1`

Explanation:

Since the vertex of the parabola is at (4,0), it has the vertex on the x axis (horizontal axis). The standard equation of an ellipse with horizontal major axis is given by:

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1`

Where (h,k) is the center of the ellipse, a is the vertex and ±√(a²- b²) is the focus (c).

Since the ellipse center is at (0, 0), h = 0 and k = 0. Also the vertex is at (4, 0) therefore a = 0

To find b we use the equation of the focus which is:

`c=√(a^2-b^2)\\ \\Substituing:\\\\3=√(4^2-b^2) \\4^2-b^2=3^2\\b^2=4^2-3^2\\b^2=16-9\\b^2=7\\b=√(7)`

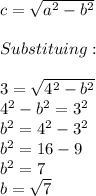
Substituting the values of a, b, h and k:

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1\\\\((x-0)^2)/(4^2)+((y-0)^2)/(√(7) ^2)=1\\\\(x^2)/(4^2)+(y^2)/(√(7) ^2)=1`