Answer:
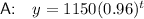
2009 = 938
Explanation:
Exponential Function
General form of an exponential function:
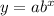
where:
- a is the initial value (y-intercept)
- b is the base (growth/decay factor) in decimal form
- x is the independent variable
- y is the dependent variable
If b > 1 then it is an increasing function
If 0 < b < 1 then it is a decreasing function
If the rate of decrease is 4% per year, that means that each year the number of fish is 96% of the previous year, since 100% - 4% = 96%
Given:
- a = 1150
- b = 96% = 0.96
- x = t = time in years
- y = total of fish
Substitute the given values into the exponential equation:
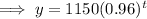
If the initial year was 2004 then:
⇒ t = 2009 - 2004 = 5
Substitute t = 5 into the equation and solve for y:
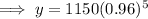
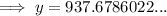
Therefore, the population in 2009 was 938 (to the nearest whole number).