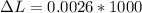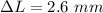Question

A plastic dowel has a Young's Modulus of 1.50 ✕ 1010 N/m2. Assume the dowel will break if more than 1.50 ✕ 108 N/m2 is exerted.

(a) What is the maximum force (in kN) that can be applied to the dowel assuming a diameter of 2.40 cm?
______Kn
(b) If a force of this magnitude is applied compressively, by how much (in mm) does the 26.0 cm long dowel shorten? (Enter the magnitude.)
mm

Answer 1

a

`F = 67867.2 \ N`

b

`\Delta L = 2.6 \ mm`

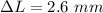
Step-by-step explanation:

From the question we are told that

The Young modulus is
`Y = 1.50 *10^(10) \ N/m^2`

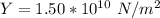
The stress is
`\sigma = 1.50 *10^(8) \ N/m^2`

The diameter is
`d = 2.40 \ cm = 0.024 \ m`

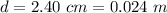
The radius is mathematically represented as

`r =(d)/(2) = (0.024)/(2) = 0.012 \ m`

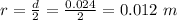
The cross-sectional area is mathematically evaluated as

`A = \pi r^2`

`A = 3.142 * (0.012)^2`

`A = 0.000452\ m^2`

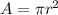
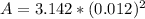
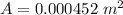
Generally the stress is mathematically represented as

`\sigma = (F)/(A)`

=>
`F = \sigma * A`

=>
`F = 1.50 *10^(8) * 0.000452`

=>
`F = 67867.2 \ N`

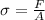
Considering part b

The length is given as
`L = 26.0 \ cm = 0.26 \ m`

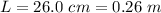
Generally Young modulus is mathematically represented as

`E = ( \sigma)/( strain )`

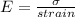
Here strain is mathematically represented as

`strain = ( \Delta L )/(L)`

So

`E = ( \sigma)/((\Delta L )/(L) )`

`E = (\sigma )/(1) * ( L)/(\Delta L )`

=>
`\Delta L = (\sigma * L )/(E)`

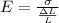
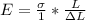
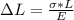
substituting values

`\Delta L = ( 1.50*10^(8) * 0.26 )/( 1.50 *10^(10 ))`

`\Delta L = 0.0026`

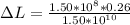
Converting to mm

`\Delta L = 0.0026 *1000`

`\Delta L = 2.6 \ mm`