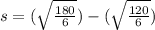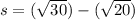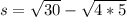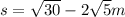Question

The formula s= I dont know how to type that but I really need helppppp​

Answer 1

`s = √(30) - 2√(5) m`

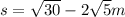
Explanation:

Given:

Formula for side length of cube,
`s = \sqrt{(SA)/(6)`

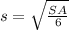
Where, S.A = surface area of a cube, and s = side length.

Required:

Difference in side length between a cube with S.A of 180 m² and a cube with S.A of 120 m²

Solution:

Difference = (side length of cube with 180 m² S.A) - (side length of cube with 120 m² S.A)

`s = (\sqrt{(180)/(6)}) - (\sqrt{(120)/(6)})`

`s = (√(30)) - (√(20))`

`s = √(30) - √(4*5)`

`s = √(30) - 2√(5) m`