Question

The length of the hypotenuse of a right triangle is 16 inches. If the length of one leg is 5 inches, what is the approximate length of the other leg? 10.5 inches 11.0 inches 15.2 inches 16.8 inches

Answer 1

Step-by-step explanation:

Hypotenuse ( h ) = 16 inches

base ( b ) = 5 inches

perpendicular ( p ) = ?

Now, Using the Pythagoras theorem

`\mathsf{{h}^(2) = {p}^(2) + {b}^(2) }`

`\mathsf{ {p}^(2) = {h}^(2) - {b}^(2) }`

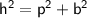
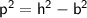
plug the values

`\mathsf{ {p}^(2) = {16}^(2) - {5}^(2) }`

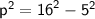
Evaluate the power

`\mathsf{ {p}^(2) = 256 - 25}`

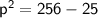
Add the numbers

`\mathsf{ {p}^(2) = 231}`

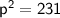
Squaring on both sides

`\mathsf{ p = 15.19}`

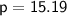
( Approximately 15.2) inches

Extra information

Pythagoras Theorem

The adjoining triangle is a right angled triangle ABC right angled at B. The side AC opposite to the right angle is called it's hypotenuse. Similarly, the sides AB and BC are called the perpendicular and the base respectively.

Pythagoras was a Greek Mathematician who loved around 500 B.C. and discovered a significant fact about right - angled triangles known as 'Pythagoras Theorem' .

The square of the hypotenuse of a right -angled triangle is equal to the sum of the squares of the remaining two sides.

( See attached picture )

Hope I helped!

Best regards!