Answer:
The answer is
x² + 3x - 4
Explanation:
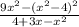
To solve the expression first factorize both the numerator and the denominator
For the numerator
9x² - ( x² - 4)²
Expand the terms in the bracket using the formula
( a - b)² = a² - 2ab + b²
(x² - 4) = x⁴ - 8x² + 16
So we have
9x² - (x⁴ - 8x² + 16)
9x² - x⁴ + 8x² - 16
- x⁴ + 17x² - 16
Factorize
that's
(x² - 16)(-x² + 1)
Using the formula
a² - b² = ( a + b)(a - b)
We have
(x² - 16)(-x² + 1) = (x + 4)(x - 4)( 1 - x)(1 + x)
For the denominator
- x² + 3x + 4
Write 3x as a difference
- x² + 4x - x + 4
Factorize
That's
- ( x - 4)(x + 1)
So we now have
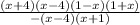
Simplify

Reduce the expression by x + 1
That's
-( x + 4)( 1 - x)
Multiply the terms
We have the final answer as
x² + 3x - 4
Hope this helps you