Answer:
B
Explanation:
(To save time, I'm going to use x instead of θ)
So we have the expression:
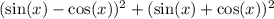
First, expand these binomials:
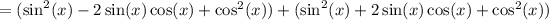
Combine like terms:

Factor out a 2:
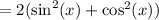
The expression inside the parentheses is the Pythagorean Identity:
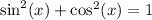
Substitute:
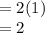
The answer is B.