Answer: At-least 89% of employees with commuting times between 54 minutes and 72 minutes .
Explanation:
Given: Commuting times for employees of a local company have a mean of 63 minutes and a standard deviation of 3 minutes.
Now, 54 minutes = (63 - 9) minutes
= (63 -3(3)) minutes
= Mean - 3 standard deviation
72 minutes = (63 + 9) minutes
=63 +3(3) minutes
= Mean + 3 standard deviation
According to Chebyshev's theorem, at least
 of the data lie within 3 standard deviations of the mean.
of the data lie within 3 standard deviations of the mean.
i.e. The percentage of employees with commuting times between 54 minutes and 72 minutes =
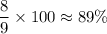
Hence, at-least 89% of employees with commuting times between 54 minutes and 72 minutes .