Question

Calculate, correct to one decimal plice

the acute angle between the lines
3x - 4y + 5 = 0 and 2x + 3y -1 = 0
A. 70.69
B. 50.2
C. 39.8
D. 19.4​

Answer 1

A. 70.69 is the correct answer.

Explanation:

Given:

Two lines:

`3x - 4y + 5 = 0 \\2x + 3y -1 = 0`

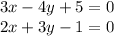
To find:

Angle between the two lines = ?

Solution:

Acute Angle between two lines can be found by using the below formula:

`tan \theta = |((m_1 - m_2))/( (1 + m_1m_2))|`

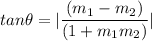
Where
`\theta` is the acute angle between two lines.

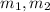`m_1, m_2` are the slopes of two lines.

Slope of a line represented by
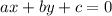`ax+by+c=0` is given as:

`m = -(a)/(b )`

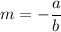
So,

`m_1 = -(3)/(- 4) = (3)/(4)`

`m_2 = -(2)/( 3)`

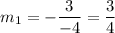
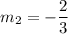
Putting the values in the formula:

`tan \theta = |(((3)/(4)- (-(2)/(3))))/( (1 + (3)/(4)* (-(2)/(3 ))))|\\\Rightarrow tan \theta = |((3)/(4)+(2)/(3))/( (1 -(1)/(2)))|\\\Rightarrow tan \theta = |((17)/(12))/( (1)/(2))|\\\Rightarrow tan \theta = (17)/(6)\\\Rightarrow \theta = tan^(-1)((17)/(6))\\\Rightarrow \theta = \bold{70.69^\circ}`

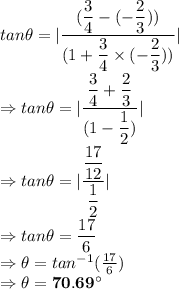
So, correct answer is A. 70.69