Answer: The length is 4 centimeters and the width is 6 centimeters.
Explanation:
If the length of the rectangle is eight centimeters less than twice the width then we could represent it by the equation L= 2w - 8 . And we know that to find the area of a rectangle we multiply the length by the width and they've already given the area so we will represent the width by w since it is unknown.
Now we know the length is 2w- 8 and the width is w so we would multiply them and set them equal to 24.
w(2w-8) = 24
2
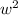 - 8w = 24 subtract 24 from both sides to set the whole equation equal zero and solve. solve using any method. I will solve by factoring.
- 8w = 24 subtract 24 from both sides to set the whole equation equal zero and solve. solve using any method. I will solve by factoring.
2
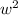 - 8w -24 = 0 divide each term by 2.
- 8w -24 = 0 divide each term by 2.
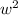 - 4w - 12 = 0 Five two numbers that multiply to get -12 and to -4
- 4w - 12 = 0 Five two numbers that multiply to get -12 and to -4
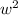 +2w - 6w - 12 = 0 Group the left hand side and factor.
+2w - 6w - 12 = 0 Group the left hand side and factor.
w(w+2) -6( w + 2) = 0 factor out w+2
(w+2)(w-6) = 0 Set them both equal zero.
w + 2 =0 or w - 6 = 0
-2 -2 + 6 +6
w= -2 or w=6
Since we are dealing with distance -2 can't represent a distance so the wide has to 6.
Now it says that the length is 8 less that twice the width.
So 2(6) - 8 = 12 -8 = 4 So the length in this care is 4.
Check.
6 * 4 = 24
24 = 24