Question

A pair of narrow, parallel slits separated by 0.230 mm is illuminated by green light (λ = 546.1 nm). The interference pattern is observed on a screen 1.50 m away from the plane of the parallel slits.

A) Calculate the distance from the central maximum to the first bright region on either side of the central maximum.
B) Calculate the distance between the first and second dark bands in the interference pattern.

Answer 1

A) y = 3.56 mm

B) y = 3.56 mm

Step-by-step explanation:

A) The distance from the central maximum to the first bright region can be found using Young's double-slit equation:

`y = (m\lambda L)/(d)`

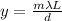
Where:

λ: is the wavelength = 546.1 nm

m: is first bright region = 1

L: is the distance between the screen and the plane of the parallel slits = 1.50 m

d: is the separation between the slits = 0.230 mm

`y = (m\lambda L)/(d) = (1*546.1 \cdot 10^(-9) m*1.50 m)/(0.230 \cdot 10^(-3) m) = 3.56 \cdot 10^(-3) m`

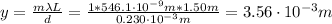
B) The distance between the first and second dark bands is:

`\Delta y = (\Delta m*\lambda L)/(d)`

Where:

`\Delta m = m_(2) - m_(1) = 2 - 1 = 1`

`\Delta y = (1*546.1 \cdot 10^(-9) m*1.50 m)/(0.230 \cdot 10^(-3) m) = 3.56 \cdot 10^(-3) m`

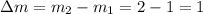
I hope it helps you!