Answer:
The value of X will be the following :

Explanation:
So as you can tell, through substitution the equation for this problem will be as follows,
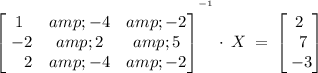
Therefore to isolate X, we have to multiply the inverse of the inverse of the co - efficient of X on either side, such that X = A
 B,
B,

To solve for X we can multiply the rows of the first matrix by the respective columns of the second matrix,
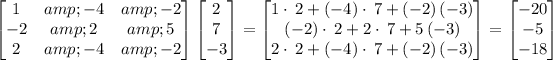
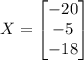 - if this matrix is matrix 1, matrix 1 will be our solution
- if this matrix is matrix 1, matrix 1 will be our solution