Question

What the answer now

Answer 1

Area of the triangle = 98.1 km²

Explanation:

In the given triangle WXV,

m∠W + m∠X + m∠V = 180°

m∠W + 119° + 34° = 180°

m∠W = 180° - 153°

m∠W = 27°

By applying Sine rule in the given triangle,

`\frac{\text{SInW}}{\text{XV}}=\frac{\text{SinX}}{\text{WV}}`

`\frac{\text{SIn27}}{\text{XV}}=\frac{\text{Sin119}}{26}`

`XV=\frac{26* (\text{Sin27})}{\text{Sin119}}`

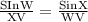
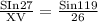
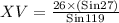
XV = 13.496 km

Area of the ΔWXV =
`(1)/(2)(\text{WV})(\text{XV})(\text{SinV})`

=
`(1)/(2)(26)(13.496)(0.55919)`

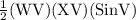
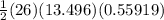
= 98.109

≈ 98.1 km²