Question

A state highway department uses a salt storage enclosure that is in the shape of a cone, as shown above. If the volume of the storage enclosure is 48π m3, then what is the diameter of the base of the cone, in meters?

Answer 1

Answer:

Explanation: it’s just right

Answer 2

Diameter of the base of the cone = 8 meters

Explanation:

State highway department uses a salt storage enclosure which is in the shape of a cone.

Height of the storage in conical shape = 9 meters

Volume of the storage = 48π m³

Let the radius of this conical storage = r meters

Formula to get the volume of a cone =
`(1)/(3)\pi r^(2)h`

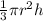
Here 'r' is the radius of the base of the cone and 'h' is the height of the cone.

Therefore, Volume =
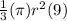
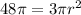
`(1)/(3)(\pi ) r^(2)(9)`

`48\pi=3\pi r^(2)`

r² =
`(48\pi )/(3\pi )`

r =
`√(16)`

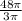
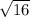
r = 4 meters

Since, diameter = 2r

= 2(4)

= 8 meters

Therefore, diameter of the base of the cone = 8 metres