Answer:
The calculated Z= 10/4.61 = 2.169
The P value is 0.975 .
Since the calculated value of z= 2.169 falls in the rejection region we therefore reject the null hypothesis at 5 % significance level . On the basis of this we conclude that the students at University A do not spend more on textbooks then the students at University B.
Explanation:
We set up our hypotheses as
H0 : x 1= x2 and Ha: x1 ≠ x2
We specify significance level ∝= 0.05
The test statistic if H0: x1= x2 is true is
Z =
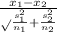
Z = 260-250/ √400/50 + 529/40
Z= 10 / √8+ 13.225
Z= 10/4.61 = 2.169
The critical value for two tailed test at alpha=0.05 is ± 1.96
The P value is 0.975 .
It is calculated by dividing alpha by 2 for a two sided test and subtracting from 1. When we subtract 0.025 ( 0.05/2)from 1 we get 0.975
Since the calculated value of z= 2.169 falls in the rejection region we therefore reject the null hypothesis at 5 % significance level . On the basis of this we conclude that the students at University A do not spend more on textbooks then the students at University B.