Question

The volume of a spherical sculpture is 256 ft³. Rhianna wants to estimate the surface area of the sculpture. To do the estimate, she approximates π using 3 in both the surface area and volume formulas for a sphere.

Using this method, what value does she get for the approximate surface area of the sculpture?

Answer 1

192

Explanation:

Answer 2

192
`ft^2`

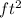
Explanation:

Given that

Volume of spherical sculpture = 256 ft³

`\pi` is used as 3.

To find:

Surface area of sculpture = ?

Solution:

First of all, let us learn about the formula for Volume and Surface Area of Sphere:

1.
`Volume =(4)/(3)\pi r^3`

2.
`Surface\ Area = 4\pi r^2`

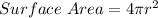
Given volume is 256 ft³.

`256 = (4)/(3)\pi r^3\\\Rightarrow 256 = (4)/(3)* 3 r^3\\\Rightarrow 256 = 4 r^3\\\Rightarrow r^3=64\\\Rightarrow \bold{r = 4\ ft}`

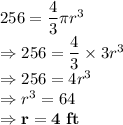
Now, let us put r = 4 in the formula of Surface Area to find the value of Surface Area:

`Surface\ Area = 4\pi 4^2 = 4 * 3 * 16 = \bold{192\ ft^2}`

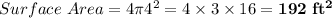
So, approximate surface area of sculpture is 192
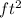`ft^2`.