Question

The perpendicular bisectors of ΔKLM intersect at point A. If AK = 25 and AM = 3n - 2, then what is the value of n?

Answer 1

n = 9 is the answer.

Explanation:

Given a Triangle
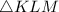`\triangle KLM` with its perpendicular bisectors intersecting at a point A.

AK = 25 units and

AM = 3n -2

To find:

Value of n = ?

Solution:

First of all, let us learn about perpendicular bisectors and their intersection points.

Perpendicular bisector of a line PQ is the line which divides the line PQ into two equal halves and is makes an angle of
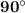`\bold{90^\circ}` with the line PQ.

And in a triangle, the perpendicular bisectors of 3 sides meet at one point and that point is called Circumcenter of the triangle.

We can draw a circle from circumcenter so that the circle passes from the three vertices of the triangle.

i.e.

Circumcenter of a triangle is equidistant from all the three vertices of the triangle.

In the given statement, we are given that A is the circumcenter of the
`\triangle KLM`.

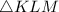Please refer to the attached image for the given triangle and sides.

The distance of A from all the three vertices will be same.

i.e. AK = AM

`\Rightarrow 25 = 3n-2\\\Rightarrow 3n =25+2\\\Rightarrow 3n =27\\\Rightarrow \bold{n = 9}`

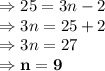
Therefore, n = 9 is the answer.