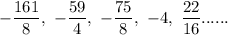Answer:
The series is given as follows;
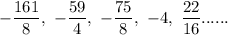
Explanation:
Assuming the series is an arithmetic progression, (AP), series, we have
The nth term of the desired series = a + (n - 1)×d
Where;
a = The first term
n = The position of the term in the series
d = The common difference
Given that the 5th term = 22/16 and the 4th term = -4, we have;
d = The difference between consecutive terms = Difference between the 5th term and the 4th term
∴ d = 22/16 - (-4) = 43/8 = 5.375
22/16 = a + (5 - 1)×5.375
∴ a = 22/16 - 4×5.375 = -20.625
The series is therefore;