Question

What are the solutions of the equation x4 – 5x2 – 14 = 0? Use factoring to solve.

Answer 1

`x=\pm 7\text{ or } x=\pm i√(2)`

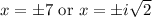
Explanation:

We have the equation:

`x^4-5x^2-14=0`

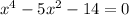
Since this is in quadratic form, we can consider using u-substitution. Thus, we will let:

`u=x^2`

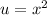
Then by substitution:

`u^2-5x-14=0`

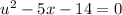
Now we can solve normally. Factor:

`(u-7)(u+2)=0`

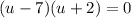
Zero Product Property:

`u=7\text{ or } u=-2`

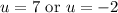
Back-substitute:

`x^2=7\text{ and } x^2=-2`

Take the square root of both sides. Since we are taking an even-root, we will need to add plus-minus:

`x=\pm 7\text{ and }x=√(-2)`

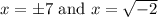
Therefore, our solutions are:

`x=\pm7\text{ and } x=\pm i√(2)`