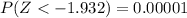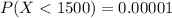Question

The distribution of baby weights at birth is left-skewed because of premies (premature babies) who have particularly low birth weights. However, within a close range of gestation times, birth weights are approximately Normally distributed. For babies born at full term (37 to 39 completed weeks of gestation), for instance, the distribution of birth weight (in grams) is approximately N(3350,440).N(3350, 440).10 Low-birth-weight babies (weighing less than 2500 grams, or about 5 pounds 8 ounces) are at an increased risk of serious health problems. Among those, very-low-birth-weight babies (weighing less than 1500 grams, or about 3 pounds 4 ounces) have the highest risk of experiencing health problems.

A. What proportion of babies born full term are low-birth-weight babies?

B. What proportion of babies born full term are very-low-birth-weight babies?

Answer 1

a

`P(X < 2500) = 0.02668`

b

`P(X < 1500) = 0.00001`

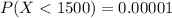
Explanation:

From the question we are told that

The population mean is
`\mu = 3350`

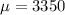
The standard deviation is
`\sigma = 440`

We also told in the question that the birth weight is approximately Normally distributed

i.e
`X \ \~ \ N(\mu , \sigma )`

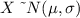
Given that Low-birth-weight babies weighing less than 2500 grams,then the proportion of babies born full term are low-birth-weight babies is mathematically represented as

`P(X < 2500) = P(( X - \mu )/(\sigma ) < (2500 - \mu)/(\sigma ) )`

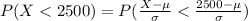
Generally

`(X - \mu)/( \sigma ) = Z (The \ standardized \ value \ of \ X )`

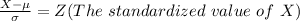
So

`P(X < 2500) = P(Z < (2500 - \mu)/(\sigma ) )`

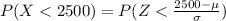
substituting values

`P(X < 2500) = P(Z < (2500 - 3350)/(440 ) )`

`P(X < 2500) = P(Z <-1.932 )`

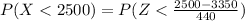
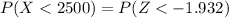
Now from the standardized normal distribution table(These value can also be obtained from Calculator dot com) the value of

`P(Z <-1.932 ) = 0.02668`

=>
`P(X < 2500) = 0.02668`

Given that very-low-birth-weight babies (weighing less than 1500 grams,then the proportion of babies born full term are very-low-birth-weight babies is mathematically represented as

`P(X < 1500) = P(( X - \mu )/(\sigma ) < (1500 - \mu)/(\sigma ) )`

`P(X < 1500) = P(Z < (1500 - \mu)/(\sigma ) )`

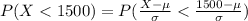
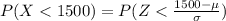
substituting values

`P(X < 1500) = P(Z < (1500 - 3350)/(440 ) )`

`P(X < 1500) = P(Z <-4.205 )`

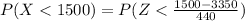
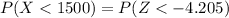
Now from the standardized normal distribution table(These value can also be obtained from calculator dot com) the value of

`P(Z <-1.932 ) = 0.00001`

`P(X < 1500) = 0.00001`