Answer:
1 year
Explanation:
Hello,
Continuously compounding with an annual interest rate of 4.5% means multiplying the initial investment by (for t tears).
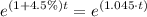
So we need to find t so that:

Rounding to the nearest whole number gives 1 year.
Hope this helps.
Do not hesitate if you need further explanation.
Thank you