Question

CAN ANYBODY HELP ME OUT

Answer 1

Correct option is

b. If two sides and one included angle are equal in triangles PQS and PRS, then their corresponding sides are also equal.

Explanation:

Here, we are given the line RQ, which is divided in two equal parts by a line PS which is perpendicular to RQ.

The foot S of PS is on the line RQ.

First of all, let us do a construction here.

Join the point R with P and P with Q.

Please refer to the attached image.

Now, let us consider the triangles PQS and PRS:

• Side QS = RS (as given)

• 
  `\angle PSR = \angle PSQ = 90^\circ`

• Side PS = PS (Common side in both the triangles)

Now, Two sides and the angle included between the two triangles are equal.

So by SAS congruence we can say that
`\triangle PRS \cong \triangle PQS`

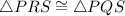
Therefore, the corresponding sides will also be equal.

RP = QP

RP is the distance between R and P.

QP is the distance between Q and P.

Hence, to prove that P is equidistant from R and Q, we have proved that:

b. If two sides and one included angle are equal in triangles PQS and PRS, then their corresponding sides are also equal.