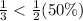Answer:
number of successes
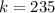
number of failure
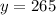
The criteria are met
A
The sample proportion is
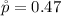
B
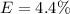
C
What this mean is that for N number of times the survey is carried out that the which sample proportion obtain will differ from the true population proportion will not more than 4.4%
Ci
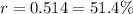

D
This 95% confidence interval mean that the the chance of the true population proportion of those that are happy to be exist within the upper and the lower limit is 95%
E
Given that 50% of the population proportion lie with the 95% confidence interval the it correct to say that it is reasonably likely that a majority of U.S. adults were happy at that time
F
Yes our result would support the claim because
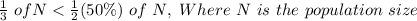
Explanation:
From the question we are told that
The sample size is
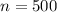
The sample proportion is
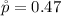
Generally the number of successes is mathematical represented as

substituting values
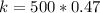
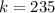
Generally the number of failure is mathematical represented as
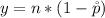
substituting values
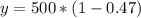
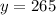
for approximate normality for a confidence interval criteria to be satisfied
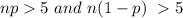
Given that the above is true for this survey then we can say that the criteria are met
Given that the confidence level is 95% then the level of confidence is mathematically evaluated as
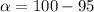
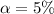
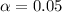
Next we obtain the critical value of
 from the normal distribution table, the value is
from the normal distribution table, the value is
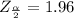
Generally the margin of error is mathematically represented as
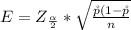
substituting values
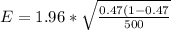
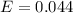
=>
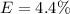
What this mean is that for N number of times the survey is carried out that the proportion obtain will differ from the true population proportion of those that are happy by more than 4.4%
The 95% confidence interval is mathematically represented as
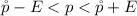
substituting values
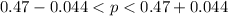
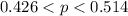
The upper limit of the 95% confidence interval is
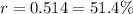
The lower limit of the 95% confidence interval is

This 95% confidence interval mean that the the chance of the true population proportion of those that are happy to be exist within the upper and the lower limit is 95%
Given that 50% of the population proportion lie with the 95% confidence interval the it correct to say that it is reasonably likely that a majority of U.S. adults were happy at that time
Yes our result would support the claim because