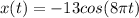Question

A buoy floating in the sea is bobbing in simple harmonic motion with amplitude 13 in and period 0.25 seconds. Its displacement d from sea level at time t=0 seconds is 0in, and initially it moves downward. (Note that downward is the negative direction.)Required:Give the equation modeling the displacement d as a function of time t.

Answer 1

The equation is
`x(t) = -13 cos (8 \pi t )`

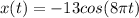
Explanation:

From the question we are told that

The amplitude is
`A = 13 \ in`

The period is
`T = 0.25`

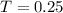
Generally the displacement function for a simple harmonic motion is mathematically represented as

`x(t) = A cos (wt )`

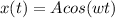
Here
`w` is the angular frequency which is mathematically represented as

`w = (2 \pi )/(T)`

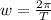
substituting values

`w = (2 \pi )/( 0.25)`

`w = 8\pi`

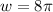
Given that at t = 0 the displacement is equal to 0 it means that there is no phase shift and also we are told that it is initially moving downward which implies that its Amplitude is
`A = -13\ in`

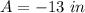
So the equation modeling the displacement d as a function of time t is mathematically represented as

`x(t) = -13 cos (8 \pi t )`