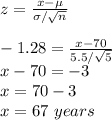Answer:
The answer is below
Explanation:
Given that:
mean (μ) = 70 years, standard deviation (σ)= 5.5 years.
a) The z score measures how many standard deviation a raw score is above or below the mean. It is given as:
 , for a sample size of n, the z score is:
, for a sample size of n, the z score is:
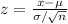
Given a sample of 5 turtles, we have to calculate the z score for x = 60 and x = 80.
For x = 60:

For x = 80:
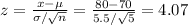
The probability that a mean life of a random sample of 5 such turtles falls between 60 and 80 years = P(60 < x < 80) = P(-4.07 < z < 4.07) = P(z < 4.07) - P(z < -4.07) = 1 - 0 = 1 = 100%
b) The z score that corresponds to top 10% is -1.28.