Answer:
a

b

Step-by-step explanation:
From the question we are told that
The distance of separation is
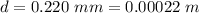
The is distance of the screen from the slit is
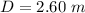
The distance between the central bright fringe and either of the adjacent bright

Generally the condition for constructive interference is

From the question we are told that small-angle approximation is valid here.
So
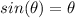
=>
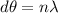
=>
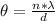
Here n is the order of maxima and the value is n = 1 because we are considering the central bright fringe and either of the adjacent bright fringes
Generally the distance between the central bright fringe and either of the adjacent bright is mathematically represented as
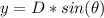
From the question we are told that small-angle approximation is valid here.
So
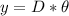
=>
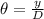
So

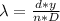
substituting values
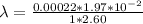
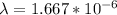

In the b part of the question we are considering the next set of bright fringe so n= 2
Hence
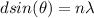
![\theta = sin^(-1)[( n * \lambda )/(d) ]](https://img.qammunity.org/2021/formulas/physics/college/7u6a3enuhjgupp2pb5oa8dvdzr6to34my3.png)
![\theta = sin^(-1)[( 2 * 1667 *10^(-9))/( 0.00022) ]](https://img.qammunity.org/2021/formulas/physics/college/9n87e9twyvmzpmwccp0iaybyhr19lfqcyt.png)
