Answer:
The area of the triangle is

Explanation:
Given
Triangle VWU
Required
Determine the Area of the Triangle
First, we'll solve for the third angle
Angles in a triangle when added equals 180; So
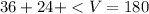
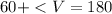
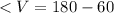
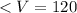
Next is to determine the length of VW using Sine Law which goes thus
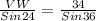 (Because 24 degrees is the angle opposite side VW)
(Because 24 degrees is the angle opposite side VW)
Multiply both sides by Sin24
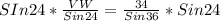
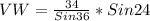
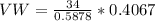
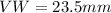 (Approximated)
(Approximated)
At this stage, we have two known sides and two known angles;
The Area can be calculated as the 1/2 * the products of two sides * Sin of the angle between the two sides
Considering VW and VU
VW = 23.5 (Calculated);
VU = 34 (Given)
The angle between these two sides is 120 (Calculated);
Hence;
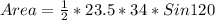
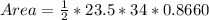
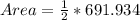
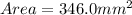
Hence, the area of the triangle is
