Question

The sum of the first 16th term of an A.P is 240 and the sum of the next 4 term is 220 find the next term of the A.P

​

Answer 1

65

Explanation:

The sum of the first 16 terms of an arithmetic progression (A.P) is 240

The sum of the next 4 terms is 220

The sum of n terms in an A.P is given by;

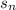`s_(n)` = n/2(2a + (n - 1)d)

240 = 8(2a + 15d) ... (i)

460 = 10(2a + 19d) .... (ii)

Simplifying this gives;

2a + 15d = 30 ... (i)

2a + 19d = 46 ... (ii)

Subtracting (i) from (ii) we get;

4d = 16

d (common difference) = 4

and a (first term) = (30 - 60)/ 2 = -15

The sequence upto 21 terms is here:

-15, -11, -7, -3, 1, 5, 9, 13, 17, 21, 25, 29, 33, 37, 41, 45, 49, 51, 55, 59, 61, 65

So the next term (21^st term) is 65.

Answer 2

Answer: a₂₁ = 65

Explanation:

The Sum of an Arithmetic Progression is the sum of the first term plus the sum of the last term divided by 2 and multiplied by the number of terms.

`a_1\ \text{is the first term}\\a_n=a_1+d(n-1)\quad \text{is the value of the nth term}\\\\`

Let's find the 16th term (n = 16)

`a_(16)=a_1+d(16-1)\\\\.\quad =a_1+15d`

Now let's find the sum of the first 16 terms. This will be Equation 1:

`S_(16)=((a_1)+(a_1+15d))/(2)* 16=240\\\\\\.\qquad 8(2a_1+15d)=240\\\\\\.\qquad 2a_1+15d=30\qquad \leftarrow \text{Equation 1}`

************************************************************************************

Repeat what we did above for the next 4 terms (n = 17 to n = 20). This will be Equation 2:

`a_(17)=a_1+d(17-1)\\\\.\quad =a_1+16d\\\\\\a_(20)=a_1+d(20-1)\\\\.\quad =a_1+19d`

`S_(17-20)=((a_1+16d)+(a_1+19d))/(2)* 4=220\\\\\\.\qquad 2(2a_1+35d)=220\\\\\\.\qquad 2a_1+35d=110\qquad \leftarrow \text{Equation 2}`

*********************************************************************************************

Now we have a system of equations. Solve using the Elimination Method:

2a₁ + 15d = 30 → -1(2a₁ + 15d = 30) → -2a₁ - 15d = -30

2a₁ + 35d = 110 → 1(2a₁ + 35d = 110) → 2a₁ + 35d = 110

20d = 80

d = 4

Input d = 4 into one the equations to solve for a₁:

Equation 1: 2a₁ + 15d = 30

2a₁ + 15(4) = 30

2a₁ + 60 = 30

2a₁ = -30

a₁ = -15

Given a₁ = -15 and d = 4, we can find the next term (n = 21)

`a_n=a_1+d(n-1)\\\\a_(21)=-15+4(21-1)\\\\.\quad =-15+4(20)\\\\.\quad = -15+80\\\\.\quad = 65`