Answer:
Explanation:
Question (1). An alloy contains zinc and copper in the ratio of 7 : 9.
If the weight of an alloy = x kgs
Then weight of copper =

=
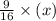
And the weight of zinc =
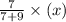
=
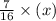
If the weight of zinc = 31.5 kg
31.5 =
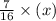
x =
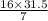
x = 72 kgs
Therefore, weight of copper =
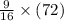
= 40.5 kgs
2). i). 2 : 3 =

4 : 5 =
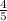
Now we will equalize the denominators of each fraction to compare the ratios.
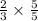 =
=

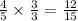
Since,

Therefore, 4 : 5 > 2 : 3
ii). 11 : 19 =
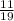
19 : 21 =
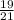
By equalizing denominators of the given fractions,

And
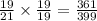
Since,

Therefore, 19 : 21 > 11 : 19
iii).
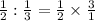
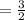
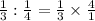
=

Now we equalize the denominators of the fractions,
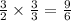
And

Since
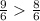
Therefore,
 will be the answer.
will be the answer.
IV).

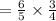

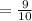
Similarly,
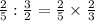
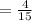
By equalizing the denominators,
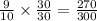
Similarly,
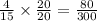
Since
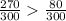
Therefore,
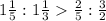
V). If a : b = 6 : 5
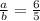
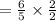
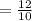
And b : c = 10 : 9
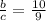
Since a : b = 12 : 10
And b : c = 10 : 9
Since b = 10 is common in both the ratios,
Therefore, combined form of the ratios will be,
a : b : c = 12 : 10 : 9