Question

all summer olympics championship distamces in the triple jump from 1896 through 2012 avereged 16.38 meters, with standard deviation of 1.34 meters. what is the probability that a randomly selected distance from the distribution would fall into each of the following intervals 16.8 meters and 17.9 meters?​

Answer 1

Answer: 0.2487

Explanation:

Given: Mean:
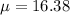`\mu=16.38` meters

Standard deviation:
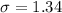`\sigma= 1.34` meters

Let X denote the distance in the triple jump.

The probability that a randomly selected distance from the distribution would fall into interval 16.8 meters and 17.9 meters =
`P(16.8<X<17.9)=P((16.8-16.38)/(1.34)<(X-\mu)/(\sigma)<(17.9-16.38)/(1.34))`

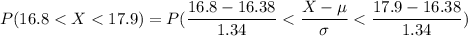
`=P(0.3134<Z<1.1343)\ \ \ [Z=(X-\mu)/(\sigma)]\\\\=P(Z<1.1343)-P(Z<0.3134)\\\\=0.8717- 0.6230\ [\text{By z-table}]\\\\=0.2487`

Hence, the probability that a randomly selected distance from the distribution would fall into interval 16.8 meters and 17.9 meters= 0.2487