Question

1. What are the formulas that help determine the equation of a circle? 2. How are the center, radius and a point on the circle expressed algebraically? 3. What do you need to know in order to use the ellipse equation formulas?

Answer 1

Answer: see below

Explanation:

1) The equation of a circle is: (x - h)² + (y - k)² = r² where

• (h, k) represents the center of the circle
• r represents the radius of the circle.

2) If you are given a point on the circle and the center (h, k)

you can input those points into the equation of a circle to find r².

Then input (h, k) and r² to identify the equation of that particular circle.

3) If you divide each term in the equation of a circle by r², you will get:

`((x-h)^2)/(r^2)+((y-k)^2)/(r^2)=1`

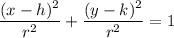
• (h, k) is the center of the circle
• r is the x-radius and y-radius

The difference between a circle and an ellipse is that an ellipse is in the shape of an oval. In other words, the x-radius and y-radius are different.

The equation of an ellipse is:

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1`

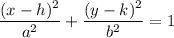
• (h, k) is the center of the ellipse
• a is the x-radius
• b is the y-radius