Question

Solve the system by substitution. x−5y=13 4x−3y=1 Enter your answer as an ordered pair (x,y).

Answer 1

(-2,-3)

Explanation:

Well in the system,

x−5y=13

4x−3y=1

We need to find x or y in either equation.

Let's do x - 5y = 13 for x.

+5y to both sides

x = 5y + 13

Now we substitute 5y + 13 for y in 4x - 3y = 1.

4(5y + 13) - 3y = 1

20y + 52 - 3y = 1

17y + 52 = 1

-52 to both sides

17y = -51

Divide all by 17

y = -3

Now we can substitute -3 for y in 4x - 3y = 1.

4x - 3(-3) = 1

4x + 9 = 1

-9 to both sides

4x = -8

Divide 4 to both sides

x = -2

Thus,

the solution is (-2,-3).

Hope this helps :)

Answer 2

( - 2 , - 3 )

Explanation:

x - 5y = 13

4x - 3y = 1

Solve the equation for x

`x - 5y = 13`

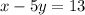
Move '5y' to R.H.S and change it's sign

`x = 13 + 5y`

Substitute the given value of X into the equation

4x - 3y = 1

`4(13 + 5y) - 3y = 1`

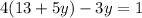
Solve the equation for y

distribute 4 through the parentheses

`52 + 20y - 3y = 1`

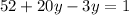
Collect like terms

`52 + 17y = 1`

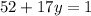
Move constant to R.H.S and change it's sign

`17y = 1 - 52`

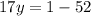
Calculate

`17y = - 51`

Divide both sides of the equation by 17

`(17y)/(17) = ( - 51)/(17)`

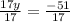
Calculate

`y = - 3`

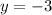
Now, substitute the given value of y into the equation

x = 13 + 5y

`x = 13 + 5 * ( - 3)`

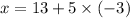
Solve the equation for x

Multiply the numbers

`= 13 - 15`

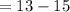
Calculate the difference

`= - 2`

The possible solution of the system is the ordered pair

( x , y )

( x , y ) = ( - 2 , - 3 )

-----------------------------------------------------------------------

Check if the given ordered pair is the solution of the system of equation

`- 2 - 5 * ( - 3) = 15`

`4 * ( - 2) - 3 * ( - 3) = 1`

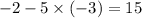
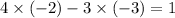
Simplify the equalities

`13 = 13`

`1 = 1`

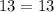
Since all of the equalities are true, the ordered pair is the solution of the system

( x , y ) = ( - 2 , - 3 )

Hope this helps..

Best regards!!